A rectangular coil of copper wires is rotated in a magnetic field

Q. No 4: Choose the correct option.

A rectangular coil of copper wires is rotated in a magnetic field. The direction of the induced current changes once in each

(a) two revolutions  (b) one revolution

(c) half revolution    (d) one-fourth revolution

Ans:

(c) When a rectangular coil of copper is rotated in a magnetic field, the direction of the induced current in the coil changes in every half rotation.

Which sources produce alternating current

Q. No 3: Which sources produce alternating current?

Ans: hydroelectric power plants, AC generators produce alternating current.

State the principle of an electric generator

Q. No 1: State the principle of an electric generator.

Ans: Electric generator works on the principle of electromagnetic induction. Electricity is generated by rotating a coil inside magnetic field.

Name some sources of direct current

Q. No 2: Name some sources of direct current.

Ans: Some sources of direct current are cell, battery and DC generator, etc.

Explain different ways to induce current in a coil

Q. No 1: Explain different ways to induce current in a coil.

Ans: The different ways to induce current in a coil are as follows:

(i) Moving a magnet towards a coil sets up a current in the coil circuit,

(ii) If a coil is moved rapidly between the two poles of a horse-shoe magnet, then an electric current is induced in the coil. 

What is the role of the split ring in an electric motor

Q. No 3: What is the role of the split ring in an electric motor?

Ans: The split ring in the electric motor also known as a commutator reverses the direction of current flowing through the coil after every half rotation of the coil. Due to this the coil continues to rotate in the same direction.

What is the principle of an electric motor

Q. No 2: What is the principle of an electric motor?

Ans:  The principle of an electric motor is based on the magnetic effect of electric current. A current-carrying loop experiences a force and rotates when placed in a magnetic field. The direction of rotation of the loop is according to the Fleming’s left-hand rule.

State Fleming’s left-hand rule

Q. No 1: State Fleming’s left-hand rule.

Ans: According to Fleming’s left hand rule if we stretch the thumb, the centre finger and the middle finger of our left hand such that they are mutually perpendicular to each other. If the centre finger gives the direction of current and middle finger points in the direction of magnetic field then the thumb points towards the direction of the force or motion of the conductor.

A positively-charged particle (alpha-particle) projected towards west is deflected towards north by a magnetic field

Q. No 3:  A positively-charged particle (alpha-particle) projected towards west is deflected towards north by a magnetic field. The direction of magnetic field is

(a) towards south  (b) towards east

(c) downward        (d) upward

Ans:

     (d) The direction of the magnetic field can be determined by the Fleming’s left hand rule. According to Fleming’s left hand rule if we stretch the thumb, the centre finger and the middle finger of our left hand such that they are mutually perpendicular to each other. If the centre finger gives the direction of current and middle finger points in the direction of magnetic field then the thumb points towards the direction of the force or motion of the conductor.
Here the direction of positively charged alpha particle is towards west this implies the direction of current is also towards west and the direction of magnetic force is towards north. Hence, according to Fleming’s left hand rule, the direction of magnetic field will be upwards.

In Activity 13.7 (page: 230), how do we think the displacement of rod AB will be affected if (i) current in rod AB is increased: (ii) a stronger horse-shoe magnet is used: and (iii) length of the rod AB is increased

Q. No 2: In Activity 13.7 (page: 230), how do we think the displacement of rod AB will be affected if (i) current in rod AB is increased: (ii) a stronger horse-shoe magnet is used: and (iii) length of the rod AB is increased?

Ans: (i) If the current in the rod is increased then rod will be deflected with greater force.

(ii) If a stronger horse-shoe magnet is used then also rod will be deflected with greater force due to the increase in magnetic field.

(iii) If the length of the rod AB is increased.

Which of the following property of a proton can change while it moves freely in a magnetic field

Q. No 1: Which of the following property of a proton can change while it moves freely in a magnetic field?

(a) mass , (b)speed ,(c) velocity, (d) momentum

Ans: velocity and momentum

Choose the correct option. The magnetic field inside a long straight solenoid-carrying current (a) is zero

Q. No 3: Choose the correct option.

The magnetic field inside a long straight solenoid-carrying current

(a) is zero

(b) decreases as we move towards its end

(c) increases as we move towards its end

(d) is the same at all points

Ans: (d) The magnetic field inside a long straight current-carrying solenoid is uniform which is represented by parallel lines. Hence it is same at all points.

The magnetic field in a given region is uniform. Draw a diagram to represent it

Q. No 2: The magnetic field in a given region is uniform. Draw a diagram to represent it.

Ans: A uniform magnetic field is represented by parallel lines

Consider a circular loop of wire lying in the plane of the table

Q. No 1: Consider a circular loop of wire lying in the plane of the table. Let the current pass through the loop clockwise. Apply the right-hand rule to find out the direction of the magnetic field inside and outside the loop.

Ans: In the following diagram the current is flowing clockwise. If we are applying right hand thumb rule to the left side of the loop then the direction of magnetic field lines inside the loop are going into the table while outside the loop they are coming out of the table. If we are applying right hand thumb rule to the right side of the loop then the direction of magnetic field lines inside the loop are again going into the table while outside the loop they are coming out of the table.

Why don’t two magnetic lines of force intersect each other

Q. No 3: Why don’t two magnetic lines of force intersect each other?

Ans: The two magnetic field lines do not intersect each other because if they do it means at the point of intersect the compass needle is showing two different directions which is not possible.

Draw magnetic field lines around a bar magnet

Q. No 1: Draw magnetic field lines around a bar magnet.

Ans: Following diagram shows the magnetic field lines around a bar magnet.

List the properties of magnetic lines of force

Q. No 2: List the properties of magnetic lines of force.

Ans: The properties of magnetic lines of forces are:

1) The direction of magnetic field lines outside the magnet sis from North Pole to South Pole.

2) The direction of magnetic field lines inside the magnet is from south pole to the north pole.

3) No two magnetic field lines intersect each other. 

Equivalent Versions of Euclid’s Fifth Postulate

Equivalent Versions of Euclid’s Fifth Postulate
‘For every line l and for every point P not lying on l, there exists a unique line m passing through P and parallel to l’.

Two distinct lines cannot have more than one point in common.

Theorem 5.1 : Two distinct lines cannot have more than one point in common.
Proof : Here we are given two lines l and m. We need to prove that they have only one point in common.
For the time being, let us suppose that the two lines intersect in two distinct points, say P and Q. So, you have two lines passing through two distinct points P and Q. But this assumption clashes with the axiom that only one line can pass through two distinct points. So, the assumption that we started with, that two lines can pass through two distinct points is wrong. From this, what can we conclude? We are forced to conclude that two distinct lines cannot have more than one point in common.

Why does a compass needle get deflected when brought near a bar magnet

Q. No 1: Why does a compass needle get deflected when brought near a bar magnet?

Ans: The needle of a compass is a small magnet. That’s why when a compass needle is brought near a bar magnet, its magnetic field lines interact with that of the bar magnet. Hence, a compass needle gets deflected.

Does Euclid’s fifth postulate imply the existence of parallel lines? Explain

1.     Does Euclid’s fifth postulate imply the existence of parallel lines? Explain

 According to Euclid's fifth postulate If a straight line falling on two straight lines makes the interior angles on the same side of it taken together less than two right angles, then the two straight lines, if produced indefinitely, meet on that side on which the sum of angles is less than two right angles.

For example

 he line PQ in Fig. 5.6 falls on lines AB and CD such that the sum of the interior angles 1 and 2 is equal to 180° on the left side of PQ. Therefore, the lines AB and CD will eventually intersect on the left side of PQ.

Euclid’s fifth postulate

How would you rewrite Euclid’s fifth postulate so that it would be easier to understand

1.How would you rewrite Euclid’s fifth postulate so that it would be easier to understand?

Solution.

There are several easy equivalent versions of Euclid's fifth postulate.

Two lines are said to be parallel if they are equidistant from one other and they do not have any point of intersection.

Then, by Play fair’s axiom (equivalent to the fifth postulate), there is a unique line m through P which is parallel to l.

Why is Axiom 5, in the list of Euclid’s axioms, considered a ‘universal truth’?

7. Why is Axiom 5, in the list of Euclid’s axioms, considered a ‘universal truth’? (Note that the question is not about the fifth postulate.)

Euclid's Axiom 5 states that "The whole is greater than the part. Since this is true for anything in any part of the world. So, this is a universal truth.

if AC = BD, then prove that AB = CD

6. In Fig. 5.10, if AC = BD, then prove that AB = CD.

From the above figure  we get that

AC     = AB + BC

BD     = BC + CD

It is given that AC = BD

Plug the value of AC we get

AB + BC      = BC + CD            … (1)

According to Euclid’s axiom,when equals are subtracted from equals, the remainders

are also equal.

Subtracting BC from both side in equation (1), we get

AB + BC − BC       = BC + CD − BC

AB     = CD

Hence proved

point C is called a mid-point of line segment AB. Prove that every line segment has one and only one mid-point

5. In Question 4, point C is called a mid-point of line segment AB. Prove that every line segment has one and only one mid-point.

Let C and D are two midpoints of the line segments AB

According to Euclid’s axioms 4

AC     = BC           ...(1)

D is also a mid point so that

AD     = DB           … (2)

We have                AB     = AB            … (3)

And  we know       AB     = AC + CB  

AB     = AD + DB

From equation (iii)

AC + CB      = AD + DB

From equation (1) and (2)plug the value of BC and DB we get

AC +AC       = AD +AD

2AC   = 2AD

Divide by 2 we get

AC     = AD

Both points are on same line so both points will superimpose and D and C are exactly at the same place

Hence midpoint of the lines segment is always unique 

If a point C lies between two points A and B such that AC = BC, then prove that AC =1/2AB. Explain by drawing the figure.

4. If a point C lies between two points A and B such that AC = BC, then prove that

AC =1/2AB. Explain by drawing the figure.

Solution

Given that C is the lies between both points A and B

And

AC     = BC

Add AC both side we get

AC  + AC     = BC + AC

According to definition of Euclid , if equals are added to equals, whole will equal 

Here, (BC + AC) coincides with AB. It is known that things which coincide with one another are equal to one another.

2AC  = AB 

Divide by 2 we get

AC     = AB/2 

Consider two ‘postulates’ given below: (i) Given any two distinct points A and B, there exists a third point C which is in between A and B. (ii) There exist at least three points that are not on the same line. Do these postulates contain any undefined terms? Are these postulates consistent? Do they follow from Euclid’s postulates? Explain.

3. Consider two ‘postulates’ given below:

(i) Given any two distinct points A and B, there exists a third point C which is in between A and B.

(ii) There exist at least three points that are not on the same line.

Do these postulates contain any undefined terms? Are these postulates consistent?

Do they follow from Euclid’s postulates? Explain.

Solutions :-

There are several undefined terms in the given postulates.

The given postulates are consistent because they refer to two different situations.

In first postulate two points A and B are given points then there will point C lying in the line between them 

Postulate (ii) states that for the given points A and B we can take point C not lying on the lines 

These postulates do not follow from Euclid’s postulates.  However they follow from the axiom, given two distinct points, there is a unique line that passes through them”.

Give a definition for each of the following terms. Are there other terms that need to be defined first? What are they, and how might you define them? (i) parallel lines (ii) perpendicular lines (iii) line segment (iv) radius of a circle (v) square

2. Give a definition for each of the following terms. Are there other terms that need to be defined first? What are they, and how might you define them?

(i) parallel lines                     (ii) perpendicular lines        (iii) line segment

(iv) radius of a circle            (v) square

Solution.

(i) parallel lines

(1) Parallel straight lines are straight lines which, being in the same plane and being produced indefinitely in both directions.

(2) Perpendicular distance between two lines is always constant

(3) Parallel lines do not meet one another in either direction.

(4) These lines never intersect each other.

(5) in below figure R and S are parallel lines and  K is the perpendicular distance

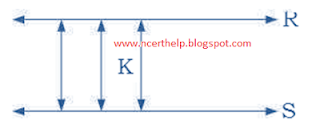 parallel lines

(ii) Perpendicular lines

These lines always intersect one another at right angle (90 degree)

In below figure P and Q are perpendicular lines 

Perpendicular lines

(iii) Line segment.

A straight line drawn from any point to any other point is called as line segment.

A line segment which extends indefinitely in both directions gives a line.

Line segment

Here PQ is a line segment 

(iv) Radius of a circle.

 A line segment joining the centre to any point on the circle is called the radius of the circle. It is the distance between the center of a circle to any point lying on the circle.

Radius of a circle

 (v) Square.

A square is that which is both equilateral and right-angled. That means all sides are equal and each angle is 90 degree.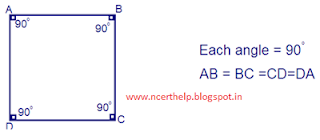 

Square

Magnetic Effects of Electric Current science 10

S.No.	Magnetic Effects of Electric Current
	Intext Question page 224
1	Why does a compass needle get deflected when brought near a bar magnet?
	Intext Question page 228
1	Draw magnetic field lines around a bar magnet.
2	List the properties of magnetic lines of force.
3	Why don’t two magnetic lines of force intersect each other?
	Intext Question page 229
1	Consider a circular loop of wire lying inthe plane of the table. Let the currentpass through the loop clockwise. Applythe right-hand rule to find out thedirection of the magnetic field insideand outside the loop.
2	The magnetic field in a given region isuniform. Draw a diagram to represent it.
3	Choose the correct option.The magnetic field inside a long straight solenoid-carrying current(a) is zero.(b) decreases as we move towards its end.(c) increases as we move towards its end.(d) is the same at all points.
	Intext Question page 231
1	Which of the following property of a proton can change while it movesfreely in a magnetic field? (There may be more than one correct answer.)(a) mass (b) speed(c) velocity (d) momentum
2	In Activity 13.7, how do we think the displacement of rod AB will beaffected if (i) current in rod AB is increased; (ii) a stronger horse-shoemagnet is used; and (iii) length of the rod AB is increased?
3	A positively-charged particle (alpha-particle) projected towards west isdeflected towards north by a magnetic field. The direction of magneticfield is(a) towards south (b) towards east(c) downward (d) upward
	Intext Question page 233
1	State Fleming’s left-hand rule.
2	What is the principle of an electric motor?
3	What is the role of the split ring in an electric motor?
	Intext Question page 236
1	Explain different ways to induce current in a coil.
	Intext Question page 237
1	State the principle of an electric generator.
2	Name some sources of direct current.
3	Which sources produce alternating current?
4	Choose the correct option.A rectangular coil of copper wires is rotated in a magnetic field. Thedirection of the induced current changes once in each(a) two revolutions (b) one revolution(c) half revolution (d) one-fourth revolution
	Intext Question page 238
1	Name two safety measures commonly used in electric circuits andappliances
2	An electric oven of 2 kW power rating is operated in a domestic electriccircuit (220 V) that has a current rating of 5 A. What result do youexpect? Explain.
3	What precaution should be taken to avoid the overloading of domesticelectric circuits?
	Exercise Questions
1	Which of the following correctly describes the magnetic field near a long straight wire? (a) The field consists of straight lines perpendicular to the wire. (b) The field consists of straight lines parallel to the wire. (c) The field consists of radial lines originating from the wire. (d) The field consists of concentric circles centred on the wire.
2	The phenomenon of electromagnetic induction is (a) the process of charging a body. (b) the process of generating magnetic field due to a current passing through a coil. (c) producing induced current in a coil due to relative motion between a magnet and the coil.
3	The device used for producing electric current is called a (a) generator. (b) galvanometer. (c) ammeter. (d) motor.
4	The essential difference between an AC generator and a DC generator is that (a) AC generator has an electromagnet while a DC generator has permanent magnet. (b) DC generator will generate a higher voltage. (c) AC generator will generate a higher voltage. (d) AC generator has slip rings while the DC generator has a commutator.
5	At the time of short circuit, the current in the circuit (a) reduces substantially. (b) does not change. (c) increases heavily. (d) vary continuously.
6	State whether the following statements are true or false. (a) An electric motor converts mechanical energy into electrical energy. (b) An electric generator works on the principle of electromagnetic induction. (c) The field at the centre of a long circular coil carrying current will be parallel straight lines. (d) A wire with a green insulation is usually the live wire of an electric supply.
7	List three sources of magnetic fields.
8	How does a solenoid behave like a magnet? Can you determine the north and south poles of a current–carrying solenoid with the help of a bar magnet? Explain.
9	When is the force experienced by a current–carrying conductor placed in a magnetic field largest?
10	Imagine that you are sitting in a chamber with your back to one wall. An electron beam, moving horizontally from back wall towards the front wall, is deflected by a strong magnetic field to your right side. What is the direction of magnetic field?
11	Draw a labelled diagram of an electric motor. Explain its principle and working. What is the function of a split ring in an electric motor?
12	Name some devices in which electric motors are used.
13	A coil of insulated copper wire is connected to a galvanometer. What will happen ifa bar magnet is (i) pushed into the coil, (ii) withdrawn from inside the coil, (iii) heldstationary inside the coil?
14	Two circular coils A and B are placed closed to each other. If the current in the coil A is changed, will some current be induced in the coil B? Give reason.
15	State the rule to determine the direction of a (i) magnetic field produced around a straight conductor-carrying current, (ii) force experienced by a current-carrying straight conductor placed in a magnetic field which is perpendicular to it, and (iii) current induced in a coil due to its rotation in a magnetic field.
16	Explain the underlying principle and working of an electric generator by drawing a labelled diagram. What is the function of brushes?
17	When does an electric short circuit occur?
18	What is the function of an earth wire? Why is it necessary to earth metallic appliances?